
Step 6 Practice More and More Calculus ProblemsĪfter you are done with understanding the formulae and theorem, it is high time for you to begin practicing on the problem. The theorem helps them in understanding how the integration and differentiation tend to be the inverse of each other. It is the fundamental theorem that helps the students in obtaining an understanding of the integration and differentiation. When it is about understanding calculus, there’s no way you can skip studying the fundamental theorem related to it. Step 5 Understand the Fundamental Theorem of Calculus This is the importance of limits here, making it highly important to understand it. This is how solving a complex function would become easy. You have to solve all the broken parts of the complex function and then sum it together. Don’t try to memorize the formula but try to understand it by going through the proof.įor the evaluation of a complex function, it is important to break that complex function into small pieces with the help of limits. You should work on understanding those formulae by going through the proof through which the formula has been obtained. The derivatives and integrals which form the calculus have some basic formula which you need to learn before getting started on calculus problem-solving. Calculus is related majorly on rate thus you need to work on learning the time vs. Rate of change can be considered as the derivative while the accumulation can be considered as integral. Calculus can be stated as the study of change, accumulation, and rate of change. Geometry: Have the knowledge about all the shapes and the properties relayed to it.īe clear with the fact that the study of calculus is divided into two parts which are differential calculus and integral calculus.Arithmetic: You should be good at the basic arithmetic and should master all the operations before seeking to understand calculus.Algebra: Lean all the algebra properties and get clear with the understanding of sets and groups.Step 1 Begin with Other Basic Parts of MathematicsĬalculus can be considered as that field of math which is further linked to various other mathematical aspects that the students should definitely work on before trying to understand calculus. Mentioned below are some steps which if effectively followed, can help in getting through calculus and allowing the students to score well in it: But calculus can be eased out if the students know the right way to work around it.

Calculus is a major part of mathematics and there’s no denying the fact that students consider it as the hardest part of mathematics too due to which most of the students struggle a lot to deal with it and understand it. Due to which we can surely call it a difficult one to deal with.
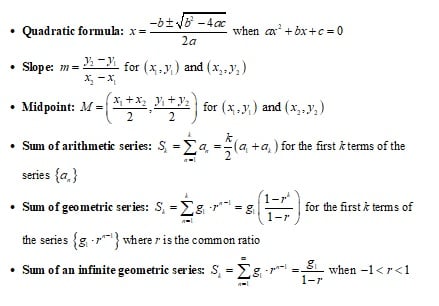
Though we can call the concepts of calculus to be brain-bending, students should have to look for ways through which they can work on this tricky subject, understand it and score well in the exams too.Ĭalculus is that branch of mathematics which consists of derivatives, limits, functions, and integrals. But the students must try and ace calculus as it is an important topic to accelerate scores. On one side calculus shows the beauty of math while on the other side it represents the agony of math too. Liberal Arts Mathematics/Quantitative Reasoning with Corequisite Support combines Liberal Arts Mathematics/Quantitative Reasoning with Math Literacy to accommodate a variety of corequisite models.Calculus is one of the subjects, with which students have a love/hate relationship. Quantitative Reasoning promotes analytical and critical thinking as well as problem-solving skills by providing coverage of prerequisite topics and real-world application topics focused on sets, logic, consumer mathematics, measurement, probability, statistics, voting, apportionment, and functions. Additional topic areas include real numbers, functions, systems of equations, geometry, and trigonometry. Liberal Arts Mathematics promotes analytical and critical thinking as well as problem-solving skills by providing coverage of prerequisite topics and traditional Liberal Arts Math topics on sets, logic, numeration, consumer mathematics, measurement, probability, statistics, voting, and apportionment. Corequisite Support for Liberal Arts Mathematics/Quantitative Reasoning provides a complete set of prerequisite topics to promote student success in Liberal Arts Mathematics or Quantitative Reasoning by developing algebraic maturity and a solid foundation in percentages, measurement, geometry, probability, data analysis, and linear functions.


 0 kommentar(er)
0 kommentar(er)
